Texas Instruments
Navigate firm data through the following pages:
| Analyst Listing | Primary Input Data |
| Derived Input Data | Valuation Model Outcomes |
Analyst Listing
The following analysts provide coverage for the subject firm as of May 2016:
| Broker | Analyst | Analyst Email |
| BMO Capital Markets | Ambrish Srivastava | ambrish.srivastava@bmo.com |
| RBC Capital Markets | Amit Daryanani | amit.daryanani@rbccm.com |
| Wedbush Securities | Betsy Van Hees | betsy.vanhees@wedbush.com |
| Evercore ISI | C.J. Muse | cj.muse@evercoreisi.com |
| Drexel Hamilton | Cody Acree | cacree@drexelhamilton.com |
| B Riley & Co | Craig A. Ellis | cellis@brileyco.com |
| Wells Fargo Securities | David Wong | david.m.wong@wellsfargo.com |
| CRT Capital Group | Douglas Freedman | dfreedman@sterneageecrt.com |
| Raymond James | J. Steven Smigie | steve.smigie@raymondjames.com |
| Credit Suisse | John Pitzer | john.pitzer@credit-suisse.com |
| Jefferies | Mark Lipacis | mlipacis@jefferies.com |
| Pacific Crest Securities-KBCM | Michael McConnell | mmcconnell@pacific-crest.com |
| Oppenheimer | Rick Schafer | rick.schafer@opco.com |
| Nomura Research | Romit Shah | romit.shah@nomura.com |
| Deutsche Bank Research | Ross Seymore | ross.seymore@db.com |
| Bernstein Research | Stacy A. Rasgon | stacy.rasgon@bernstein.com |
| Cowen & Company | Timothy Arcuri | timothy.arcuri@cowen.com |
| Stifel Nicolaus | Tore Svanberg | tsvanberg@stifel.com |
| Mizuho Securities USA | Vijay Rakesh | vijay.rakesh@us.mizuho-sc.com |
| SunTrust Robinson Humphrey | William Stein | william.stein@suntrust.com |
| Daiwa Securities Co. Ltd. | Yoko Yamada | yoko.yamada@us.daiwacm.com |
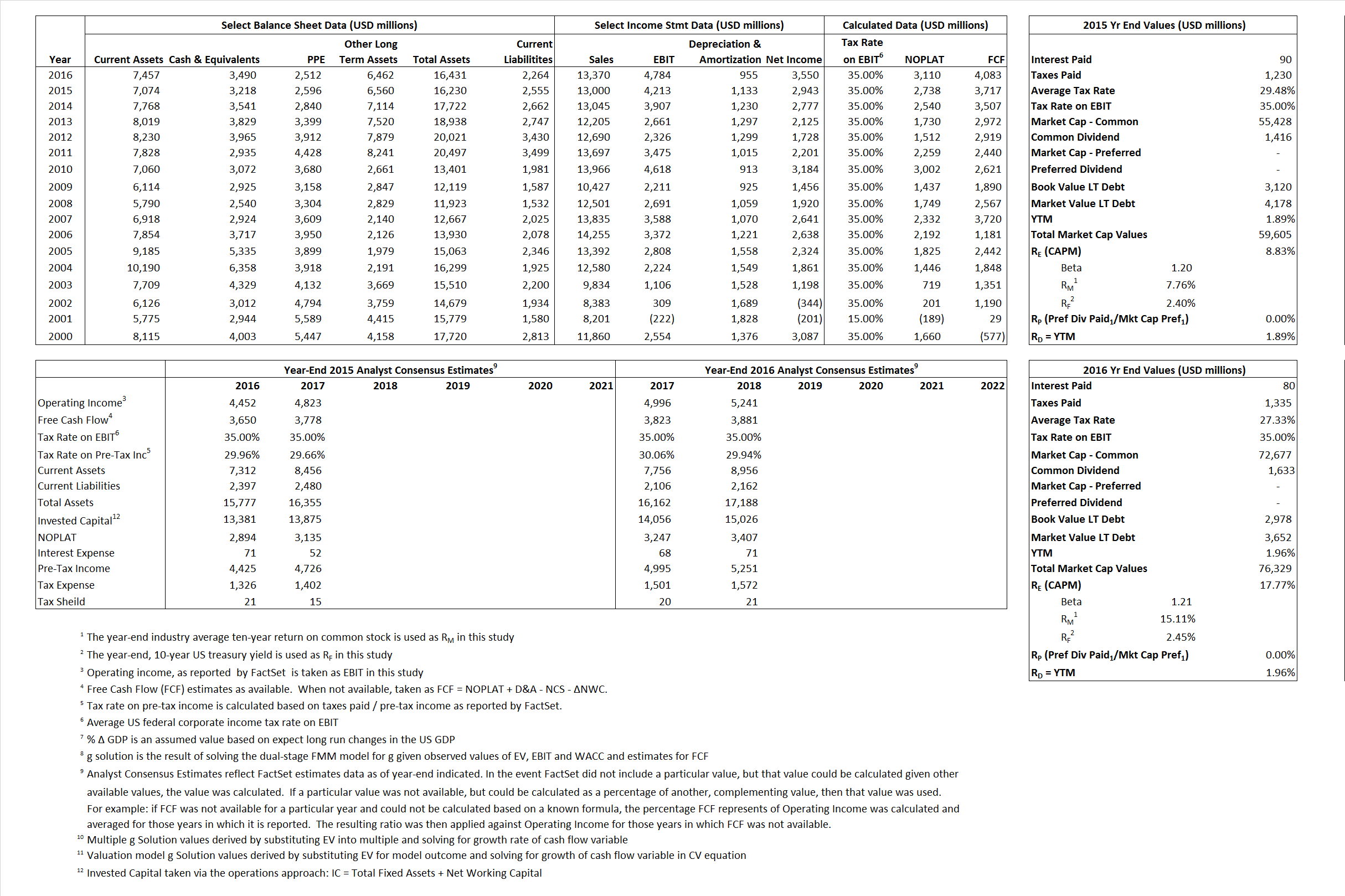
Primary Input Data
Derived Input Data
Derived Input |
Label |
2015 Value
|
2016 Value
|
Equational Form |
| Net Operating Profit Less Adjusted Taxes | NOPLAT | 2,738 | 3,110 | |
| Free Cash Flow | FCF | 3,717 | 4,083 | |
| Tax Shield | TS | 27 | 22 | |
| Invested Capital | IC | 13,675 | 14,167 | |
| Return on Invested Capital | ROIC | 20.03% | 21.95% | |
| Net Investment | NetInv | (252) | 1,447 | |
| Investment Rate | IR | -9.20% | 46.53% | |
| Weighted Average Cost of Capital | WACCMarket | 8.31% | 16.99% | |
| WACCBook | 10.21% | 9.95% | ||
| Enterprise value | EVMarket | 56,387 | 72,839 | |
| EVBook | 55,058 | 72,165 | ||
| EV/EBIT Multiple | 13.38 | 15.23 | ||
| Long-Run Growth | g = IR x ROIC |
-1.84% | 10.21% | Long-run growth rates of the income variable are used in the Continuing Value portion of the valuation models. |
| g = % |
2.50% | 2.50% |
Valuation Model Outcomes
The outcomes presented in this study are the result of original input data, derived data, and synthesized inputs and, depending on the equational form of any particular valuation model, may result in irrelevant or implausible results. For example, in the event WACC < g, the value of this term, often found in the denominator of an equation’s continuation value term, will be expressly negative and may result in a negative overall valuation for the firm. In the event of a WACC < g relation, the model form as applied to the subject firm offers an irrelevant outcome.
Valuation Model Type |
Label |
Equational form
|
|
| Key Value Driver (NOPLAT) | KVD (NOPLAT) | ||
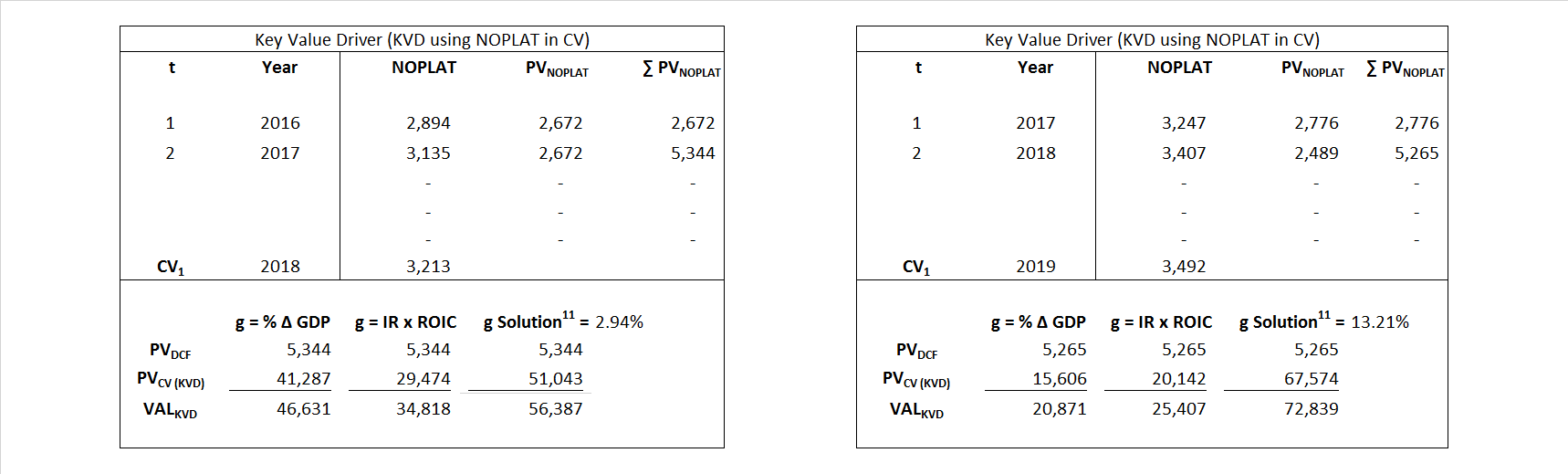 |
|||
| Key Value Driver (FCF) | KVD (FCF) |
|
|
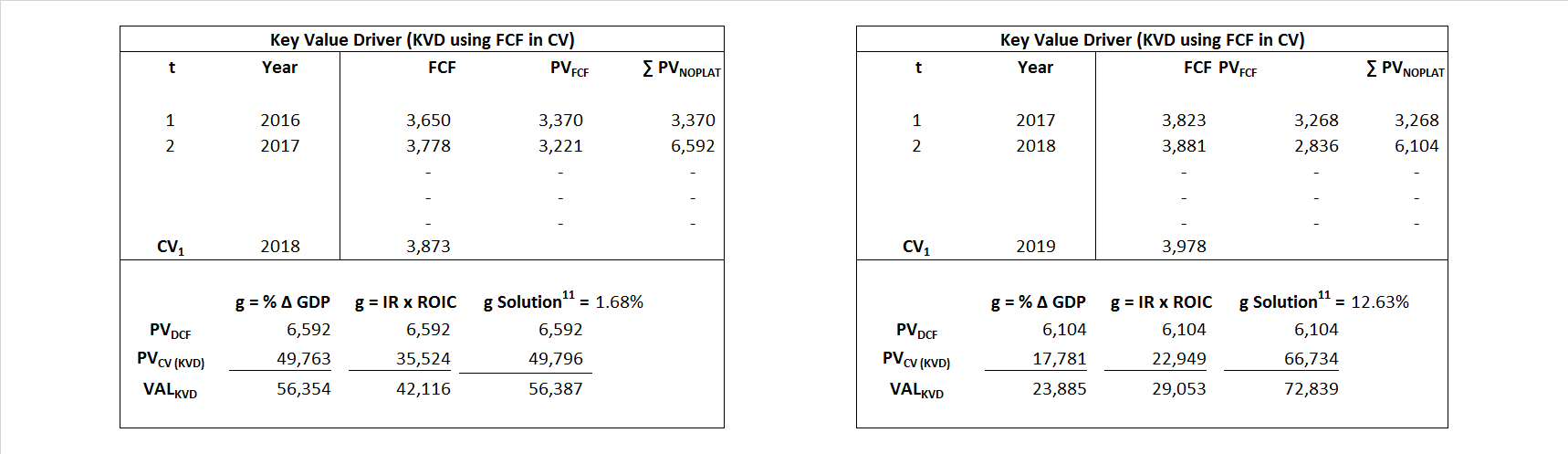 |
|||
| Free Cash Flow | FCF | ||
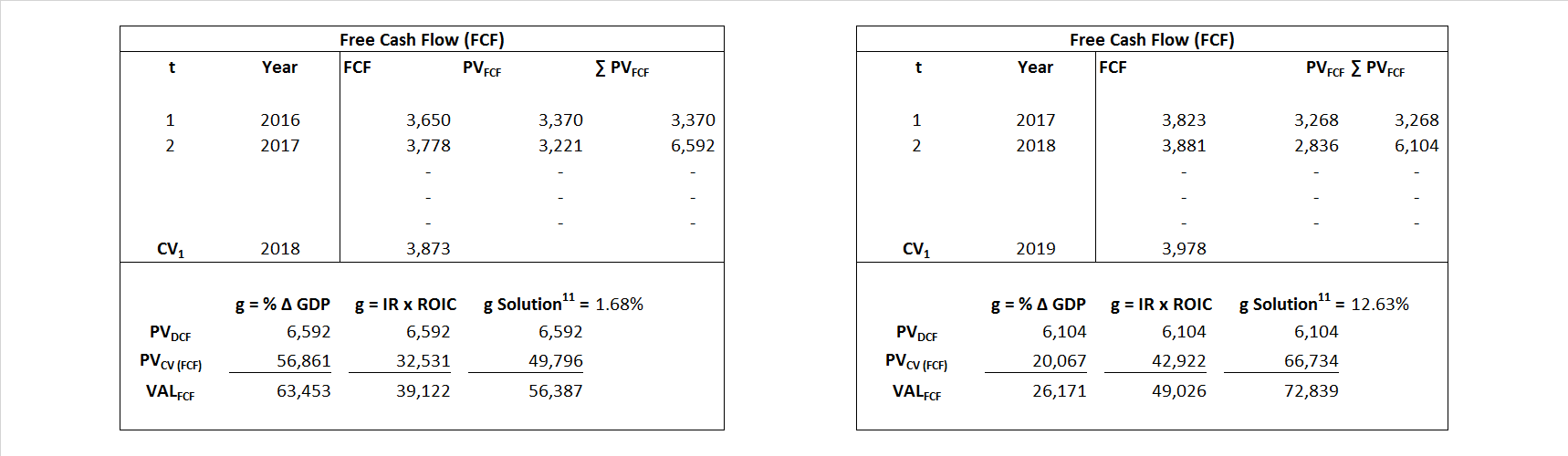 |
|||
| Economic Profit | ECON π | ||
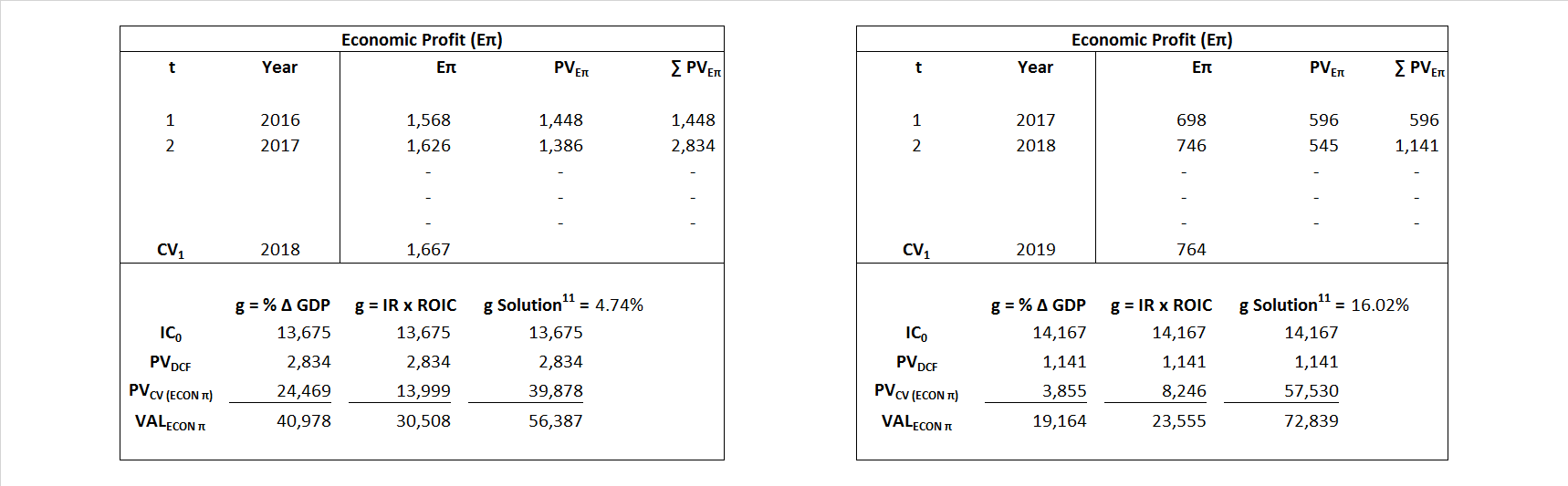 |
|||
| Adjusted Present Value | APV | ||
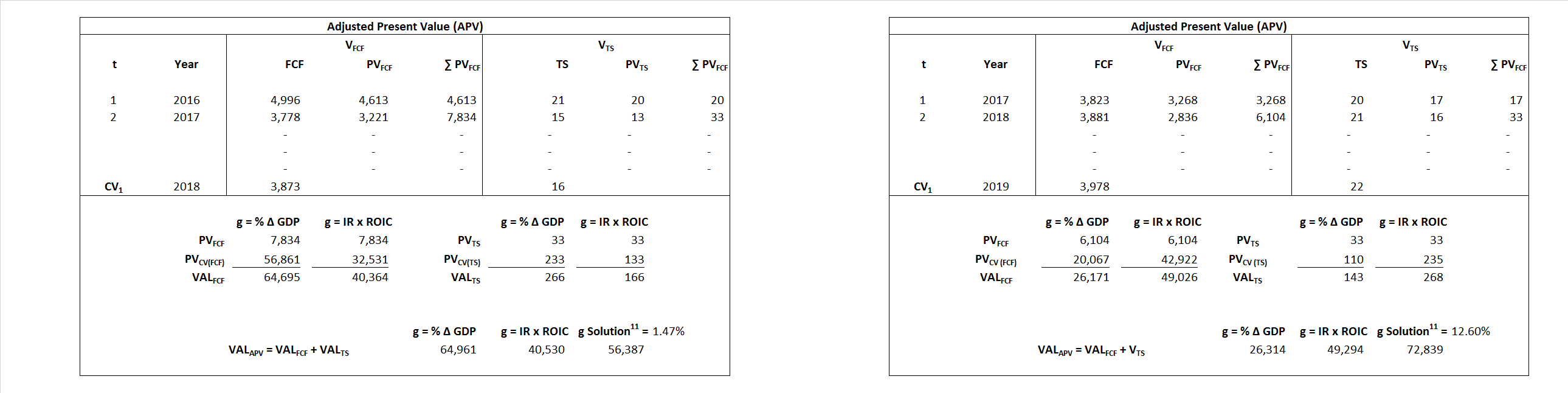 |
|||
| Forward Market Multiple | FMM | ||
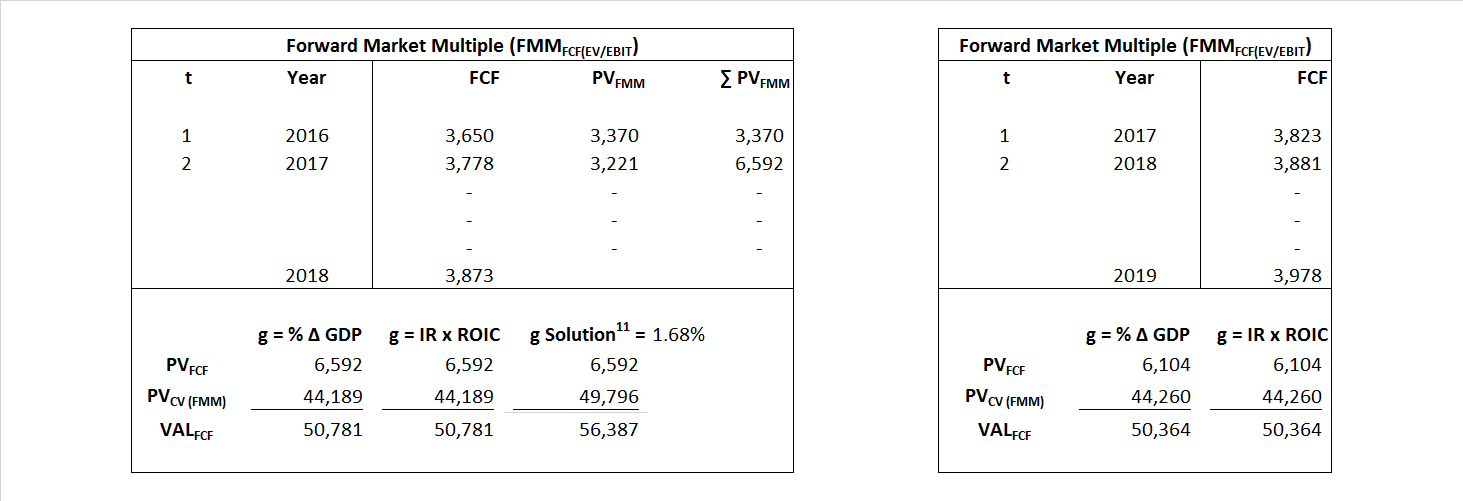 |
|||